For a process characteristic with mean μ and standard deviation σ, the population-based capability indices are defined as follows. For sample observations, the parameters are replaced by their estimates:
LSL = Lower specification limit
USL = Upper specification limit
T = Target value
For estimates of Within Sigma capability, σ is estimated using the subgrouping method that you specified. For estimates of Overall Sigma capability, σ is estimated using the sample standard deviation. If either of the specification limits is missing, the capability indices containing the missing specification limit are reported as missing.
The 100(1 - α)% confidence interval for Cp is calculated as follows:
df is the degrees of freedom
N is the number of observations
For Within Sigma capability with unbalanced subgroups, the degrees of freedom is equal to N - m, where m is the number of subgroups.
For Within Sigma capability with balanced subgroups, the degrees of freedom calculation depends on the within sigma estimation method. When Within Sigma is estimated by the average of the unbiased standard deviations, N - m is multiplied by a scale factor between 0.875 and 1. See Bissell (1990). When Within Sigma is estimated by the average of ranges, the degrees of freedom is calculated using a formula based on the subgroup sample size. See David (1951).
The 100(1 - α)% confidence interval for Cpk is calculated as follows:
N is the number of observations
df is the degrees of freedom
For Within Sigma capability with unbalanced subgroups, the degrees of freedom is equal to N - m, where m is the number of subgroups.
For Within Sigma capability with balanced subgroups, the degrees of freedom calculation depends on the within sigma estimation method. When Within Sigma is estimated by the average of the unbiased standard deviations, N - m is multiplied by a scale factor between 0.875 and 1. See Bissell (1990). When Within Sigma is estimated by the average of ranges, the degrees of freedom is calculated using a formula based on the subgroup sample size. See David (1951).
The 100(1 - α)% confidence interval for Cpm is calculated as follows:
N is the number of observations
T is the target value
s is the sigma estimate
For Overall Sigma capability, s is the Overall Sigma estimate. For Within Sigma capability, s is replaced by the Within Sigma estimate.
Lower and upper confidence limits for Cpl and Cpu are computed using the method of Chou et al. (1990).
The 100(1 - α)% confidence limits for Cpl (denoted by CPLL and CPLU) satisfy the following equations:
tn-1(δ) has a non-central t-distribution with n - 1 degrees of freedom and noncentrality parameter δ
The 100(1 - α)% confidence limits for Cpu (denoted by CPUL and CPUU) satisfy the following equations:
tn-1(δ) has a non-central t-distribution with n - 1 degrees of freedom and noncentrality parameter δ
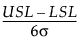



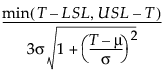
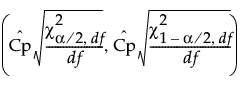
 is the estimated value for Cp
is the estimated value for Cp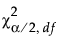 is the (
is the (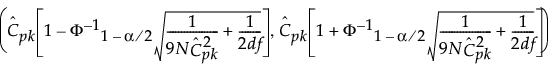
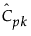 is the estimated value for Cpk
is the estimated value for Cpk is the (1 -
is the (1 - 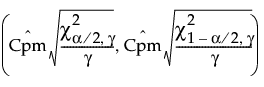
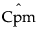 is the estimated value for Cpm
is the estimated value for Cpm is the (
is the (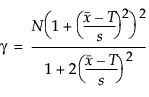
 is the mean of the observations
is the mean of the observations where
where 
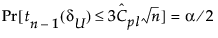 where
where 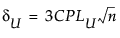
 is the estimated value for Cpl
is the estimated value for Cpl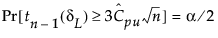 where
where 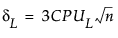
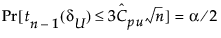 where
where 
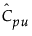 is the estimated value for Cpu
is the estimated value for Cpu