Publication date: 05/05/2023
Response Surface Designs
Response surface designs are useful for modeling quadratic surfaces. A response surface model can identify a point where a minimum or maximum value of the response occurs, if one exists inside the design region. Three distinct values for each factor are necessary to fit a quadratic function, so standard two-level designs are not appropriate for fitting curved surfaces.
Response surface designs are capable of fitting a second-order prediction equation for the response. The quadratic terms in these equations model the curvature in the true response function. If a maximum or minimum exists inside the design region, the point where that value occurs can be estimated.
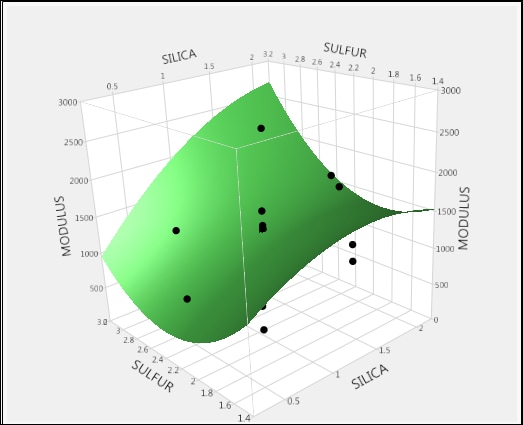
Figure 12.1 Model for Response Surface Design Results
Contents
Overview of Response Surface Designs
Example of a Response Surface Design
Build a Response Surface Design
Responses
Factors
Choose a Design
Output Options
Make Table
Response Surface Design Options
Want more information? Have questions? Get answers in the JMP User Community (community.jmp.com).