 Formulas for Metrics
Formulas for Metrics
Coverage and diversity are two metrics calculated for covering array designs. The formulas for coverage and diversity depend on whether there are combination constraints. The following notation is used:
uCv is the number of combinations of u things taken v at a time
t is the strength of the design
K is the number of factors
M = KCt
i = 1, 2,..., M is an index that orders all combinations, or projections, of t factors
vik is the number of levels for the kth factor
ni is the number of distinct t tuples in the design for the ith projection
pi is the product of the vik for the factors in the ith projection
r is the number of runs in the design
 Unconstrained Design
Unconstrained Design
Coverage and Diversity are given by the following:
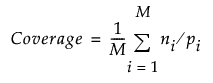

 Constrained Design
Constrained Design
In a constrained design, certain t tuples are not allowed. This can result in missing values for some t tuples. For some combinations of t factors, there might be no valid t tuples whatsoever. Coverage and diversity must be defined in terms of the possible valid combinations. For this reason, the formulas for constrained designs require additional notation:
ai is the number of invalid t tuples arising from factors in the ith projection
m is the number of projections where there are no valid t tuples
qi is the number of runs in the design with missing values for any factor in the ith projection
ri = r - qi
M ′ = M - m
Coverage and Diversity are given by the following:
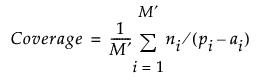
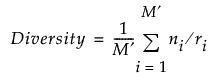
If there are no invalid t tuples (M′ = M) and if there are no missing values (ri = r, for all i), then the definitions for coverage and diversity for constrained designs reduce to the definitions for unconstrained designs. See Morgan (2014).