Fractional Increase in CI Length
In the Evaluate Design report, the Fractional Increase in CI Length is reported. The fractional increase in CI length compares the length of a parameter’s confidence interval as given by the current design to the length of such an interval given an ideal design:
• The length of the ideal confidence interval for the parameter is subtracted from the length of its actual confidence interval.
• This difference is then divided by the length of the ideal confidence interval.
For an orthogonal D-optimal design, the fractional increase is zero. In selecting a design, you would like the fractional increase in confidence interval length to be as small as possible.
The Ideal Design
The covariance matrix for the ordinary least squares estimator is σ2(X′X)-1. The diagonal elements of (X′X)-1 are the relative variances (the variances divided by σ2) of the parameter estimates. For two-level designs and using the effects coding convention (see Coding), the minimum value of the relative variance for any parameter estimate is 1/n, where n is the number of runs. This occurs when all the effects for the design are orthogonal and the design is D-optimal.
Let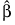 
denote the vector of parameter estimates. The ideal design, which might not exist, is a design whose covariance matrix is given as follows:
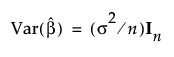
where In is the n by n identity matrix and σ is the standard deviation of the response.
If an orthogonal D-optimal design exists, it is the ideal design. However, the definition above extends the idea of an ideal design to situations where a design that is both orthogonal and D-optimal does not exist.
The definition is also appropriate for designs with multi-level categorical factors. The orthogonal coding used for categorical factors allows such designs to have the ideal covariance matrix. For a Custom Design, you can view the coding matrix by selecting Save X Matrix from the options in the Custom Design window, making the design table, and looking at the script Model Matrix that is saved to the design table.
Fractional Increase in Length of Confidence Interval
Note that, in the ideal design, the standard error for the parameter estimates would be given as follows:
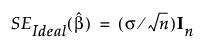
The length of a confidence interval is determined by the standard error. The Fractional Increase in Confidence Interval Length is the difference between the standard error of the given design and the standard error of the ideal design, divided by the standard error of the ideal design.
Specifically, for the ith parameter estimate, the Fractional Increase in Confidence Interval Length is defined as follows:
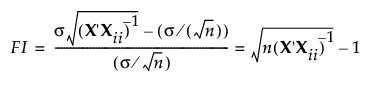
where
σ2 is the unknown response variance,
X is the model matrix for the given design, defined in The Alias Matrix,
is the ith diagonal entry of 
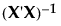, and
n is the number of runs.