Margin of Error for One Sample Mean
Use the Margin of Error for One Sample Mean Explorer to determine a sample size for a confidence, prediction, or tolerance interval for one sample mean. Select DOE > Sample Size Explorers > Confidence Intervals > Margin of Error for One Sample Mean. Explore the trade offs between variability assumptions, sample size, significance, and the margin of error.
Interval Explorer for One Sample Mean Settings
Set study assumptions and explore sample sizes using the radio buttons, text boxes, and menus. The profiler updates as you make changes to the settings. Alternatively, change settings by dragging the cross hairs on the profiler curves.
Interval Type
One-sided
Specifies a one-sided interval (upper or lower bound)
Two-sided
Specifies a two-sided interval.
Interval Purpose
Confidence
Specifies a confidence interval for a mean.
Prediction
Specifies a prediction interval for one future observation.
Tolerance
Specifies a tolerance interval to cover a proportion of the population.
Preliminary Information
Alpha
Specifies the confidence level is 1 - alpha. The default alpha level is 0.05 for a 95% confidence interval.
Proportion
(Available only when Tolerance is selected for Interval Purpose.) Specifies the proportion of the population for the tolerance interval to cover.
Population Standard Deviation
Specifies the distribution for calculations.
Yes
Specifies a known standard deviation, calculations use the z distribution.
No
Specifies an unknown standard deviation, calculations use the t distribution.
Interval Explorer for One Sample Mean Profiler
The profiler enables you to visualize the impact of sample size assumptions on the margin of error calculations.
Solve for
Enables you to solve for the sample size or assumed standard deviation.
Margin of Error
Specifies the half-width of the interval. With all other parameters fixed, margin of error decreases as sample size increases.
Sample Size
Specifies the total number of observations (runs, experimental units, or samples) needed to construct your interval.
Std Dev (σ)
Specifies the assumed population standard deviation.
Interval Explorer for One Sample Mean Options
The Explorer red triangle menu and report buttons provide additional options:
Simulate Data
Opens a data table of simulated data based on the explorer settings. View the simulated response column formula for the settings used.
Make Data Collection Table
Creates a new data table that you can use for data collection. The table includes scripts to facilitate data analysis.
Save Settings
Saves the current settings to the Saved Settings table. This enables you to save a set of alternative study plans. See Saved Settings in the Sample Size Explorers.
Reset to Defaults
Resets all parameters and graphs to their default settings.
Help
Opens JMP online help.
Statistical Details for the Margin of Error for One Sample Mean Explorer
The calculation for each interval type uses the standard normal-based procedures if σ is known and t distribution procedures or approximations otherwise.
Confidence Intervals
The margin of error (MOE) for a two-sided confidence intervals is calculated as follows:
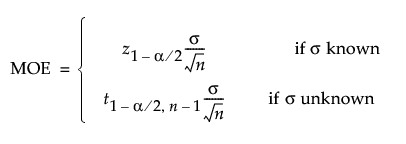
The MOE for a one-sided confidence interval is calculated as follows:
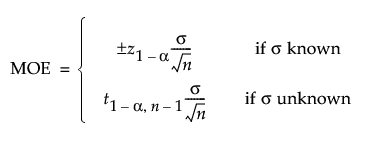
where:
α is the significance level
z1-α is the (1 - α)th quantile of the normal distribution
t1-α,ν is the (1 - α)th quantile of the central t-distribution with ν degrees of freedom
σ is the assumed population standard deviation
n is the number of samples.
Prediction Intervals
The margin of error (MOE) for prediction intervals is calculated as follows:
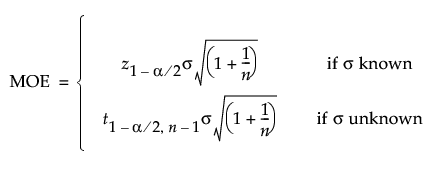
The bound is calculated as follows:
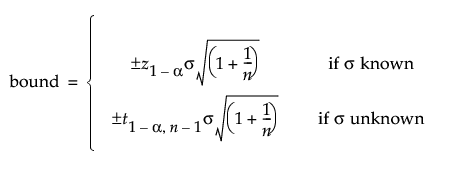
where:
α is the significance level
z1-α is the (1 - α)th quantile of the normal distribution
t1-α,ν is the (1 - α)th quantile of the central t-distribution with ν degrees of freedom
σ is the assumed population standard deviation
n is the number of samples.
Tolerance Intervals
When the population standard deviation is known, the margin of error is computed based on approximate procedures described in Krishnamoorthy and Mathew (2009). When the population standard deviation is unknown, the two-sided interval uses a correction to Howe’s approximation.
For a two-sided tolerance interval to cover proportion q of the population the margin of error (MOE) for tolerance intervals is calculated as follows:
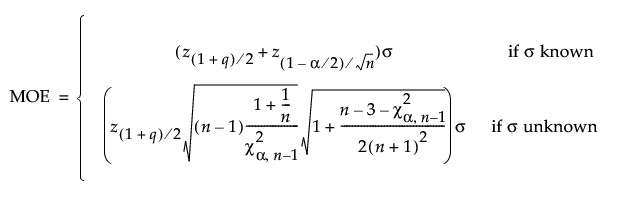
For a one-sided tolerance interval to cover proportion q of the population the margin of error (MOE) for tolerance intervals is calculated as follows:
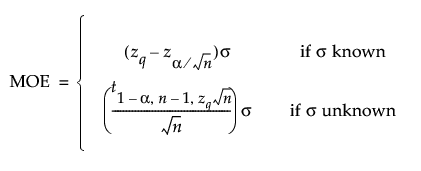
where:
α is the significance level
zα is the αth quantile of the normal distribution
χ2α,ν is the αth quantile of a central χ2 distribution with ν degrees of freedom
t1-α,ν is the (1 - α)th quantile of the central t-distribution with ν degrees of freedom
σ is the assumed population standard deviation
n is the number of samples.