Example Comparison of Sphere-Packing, Latin Hypercube, and Uniform Methods

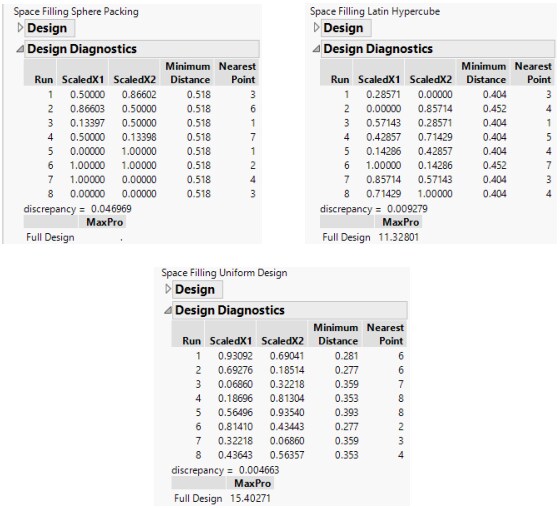
To compare space-filling design methods, create the Sphere Packing, Latin Hypercube, and Uniform designs, as shown in the examples. The Design Diagnostics tables show the values for the factors scaled from zero to one. The minimum distance is based on these scaled values and is the minimum distance from each point to its closest neighbor. The discrepancy value is the integrated difference between the design points and the uniform distribution.

Figure 22.12 shows a comparison of the design diagnostics for three eight-run space-filling designs. Note that the discrepancy for the Uniform design is the smallest (best). The discrepancy for the Sphere-Packing design is the largest (worst). The discrepancy for the Latin Hypercube takes an intermediate value that is closer to the optimal value. The MaxPro criteria is smaller for the Latin Hypercube design as compared to the Uniform Design.

Also note that the minimum distance between pairs of points is largest (best) for the Sphere-Packing method. The Uniform design has pairs of points that are only about half as far apart. The Latin Hypercube design behaves more like the Sphere-Packing design in spreading the points out.

For both spread and discrepancy, the Latin Hypercube design represents a compromise design.

Figure 22.12 Comparison of Diagnostics for Three Eight-Run Space-Filling Methods