Example of the Fit Definitive Screening Platform
Use the Fit Definitive Screening platform to evaluate a design with 18 runs. The design is a definitive screening design for six factors in two blocks with 4 extra runs.
Note: For an example of a design with continuous and categorical factors, open the sample data file Peanut Data.jmp and run the Fit Definitive Screening table script.
Fit the Model
1. Select Help > Sample Data Folder and open Design Experiment/Extraction 3 Data.jmp.
2. Select DOE > Definitive Screening > Fit Definitive Screening.
3. Select Yield and click Y.
4. Select Lot through Time and click X.
5. Click OK.
The fit performs a two-stage analysis. For more information about the algorithm, see Statistical Details for the Fit Definitive Screening Platform.
Stage 1: Main Effect Estimates
Stage 1 determines which main effects are likely to be active.
Figure 9.2 Stage 1 Report for Main Effects
Note: The fake factors do not appear in the design or as factors in the analysis.
A two-degree-of-freedom error sum of squares is computed from the four runs corresponding to the two fake factors. Because the fake factors are, by construction, inactive, this estimate of error variance is unbiased. For each main effect, the main effects response YME is tested against this estimate. In this example, three factors, Methanol, Ethanol, and Time, have p-values smaller than the threshold value and are retained as active. For more information about the threshold values, see Stage 1 Methodology.
The variability from the three inactive factors, Propanol, Butanol, and pH, is pooled with the fake factor sum of squares to produce the five-degree-of-freedom RMSE statistic shown in Figure 9.2.
Stage 2: Even Order Effect Estimates
Stage 2 uses guided subset selection to arrive at a list of second-order effects that are likely to be active. Interactions and quadratic terms are second-order or even order effects.
Figure 9.3 Stage 2 Report for Even-Order Effects
Because three main effects are identified as active in Stage 1, the guided subset selection procedure for active second-order effects can continue until all second-order effects are included. Because all six second-order effects are reported in Stage 2, it follows that the Stage 2 RMSE remained larger than the Stage 1 RMSE. See Stage 2 Methodology.
The two-degree-of-freedom RMSE given in the Stage 2 report is the error estimate obtained from the final subset of all six second-order effects.
Combined Results
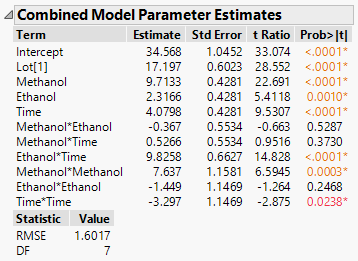
The effects selected for the model are listed in the Combined Model Parameter Estimates report.
Figure 9.4 Combined Model Parameter Estimates Report
The RMSE and degrees of freedom given at the bottom of the report are the usual standard least squares quantities. Use these effects as potential factors for your final model.
Reduce the Model
The Make Model button enters the model for the listed terms in a Fit Model specification window. To run the model directly using standard least squares, click the Run Model button.
1. Click Run Model.
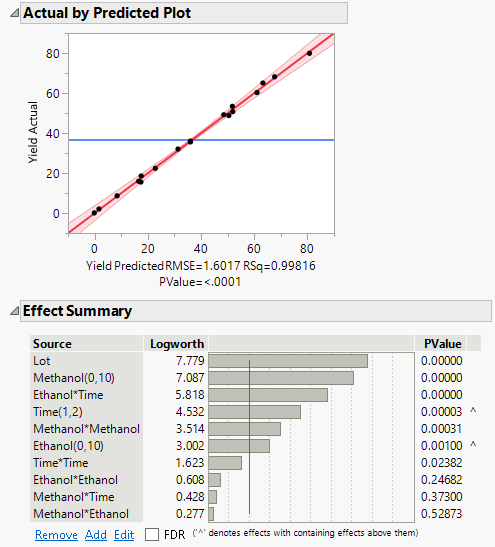
The Actual by Predicted Plot shows no lack of fit. The Effect Summary report suggests that you can reduce the model further.
Figure 9.5 Actual by Predicted Plot and Effect Summary Report
2. Select Methanol*Ethanol in the Effect Summary report and click Remove.
Methanol*Time has p-value 0.33750. Remove it next.
3. Select Methanol*Time in the Effect Summary report and click Remove.
Ethanol*Ethanol has p-value 0.15885. Remove it next.
4. Select Ethanol*Ethanol in the Effect Summary report and click Remove.
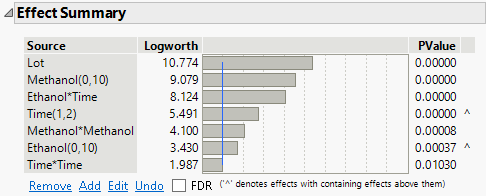
Figure 9.6 Effect Summary Report Showing Effects in Final Model
The remaining effects are significant. You conclude that these are the active effects.