Stability Analysis in the Degradation Platform
Stability analysis is used in setting pharmaceutical product expiration dates. Three linear degradation models are fit, and an expiration date is estimated following International Conference on Harmonisation (ICH) guidelines. The ICH guidelines are used for the general framework of determining if batches can be pooled for expiration dating (ICH Q1E 2003). For specific implementation details, see the STAB macro and FDA guidelines in Chow (2007, Appendix B). See Example of Stability Analysis.
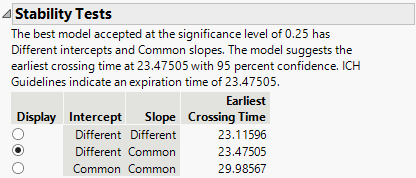
The Stability Tests report summarizes three degradation models and their corresponding earliest crossing times. The best model is selected and displayed in the Overlay plot. The models are listed in the order of complexity.
The first model fits different intercepts and different slopes to each batch. (In the procedure steps and model comparisons described below, this is the full model.) When this model is used for estimating the expiration date, the mean square error (MSE) is not pooled across batches. Confidence intervals are computed for each batch using individual mean squared errors, and the interval that crosses the specification limit first is used to estimate the expiration date. The earliest crossing times are based on 95% two-sided confidence intervals when there are two specification limits provided; the earliest crossing times are based on 95% one-sided confidence intervals when there is only one specification limit provided.
Note: When the Use Pooled MSE for Nonpoolable Model option is selected, the first model uses a model with a pooled mean square error (MSE) to compute the earliest crossing time. See Statistical Details for Stability Analysis in the Degradation Platform.
The second model fits different intercepts to each batch, but fits a common slope across all batches. The third model fits a common intercept and a common slope across all batches. When this model is appropriate, it provides the expiration date farthest into the future.
Figure 16.19 Stability Tests Report
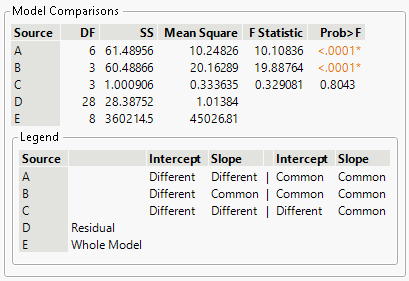
The Model Comparisons section of the Stability Tests report summarizes the tests of significance for each of the stability models. The Legend describes each source. The procedure for determining the best model for expiration dating considers the p-values for Sources C and B. The sources are listed below in reverse order to accommodate the order of steps in the procedure.
Tip: The ICH guidelines specify using a significance level of 0.25 to determine the appropriate model. You can follow the steps below and compare the p-values at each source to a different significance level. If this results in a different model being selected, you can use the radio buttons in the Display column of the table in the Stability Tests summary report.
Source E
Specifies the sum of squared responses minus the Source D sum of squares. The value in the Mean Square column for Source E is the Source E SS value divided by the Source E degrees of freedom, which are equal to the number of parameters in the full model (different intercepts and different slopes).
Source D
Specifies the error sum of squares and corresponding MSE for the full model (different intercepts and different slopes).
Source C
Specifies the test of equal slopes. This is a test of the second model (different intercepts and common slope) versus the full model (different intercepts and different slopes).
– If the p-value is less than 0.25, the slopes are assumed to be different across batches. The procedure stops and the full model (different intercepts and different slopes) is used to estimate the expiration date.
– If the p-value is greater than or equal to 0.25, the slopes are assumed to be common across batches and you then evaluate the intercepts using Source B.
Source B
Specifies the test for equal intercepts. This is a test of the third model (common intercepts and common slopes) versus the second model (different intercepts and common slope).
– If the p-value is less than 0.25, the intercepts are assumed to be different across batches, and the second model (different intercepts and common slope) is used to estimate the expiration date.
– If the p-value is greater than or equal to 0.25, the intercepts are assumed to be common across batches, and the third model (common intercepts and common slopes) is used to estimate the expiration date.
Source A
Specifies the test of the third model (common intercepts and common slopes) versus the full model (different intercepts and different slopes). This test is not used in the procedure to determine the best model for expiration dating.
Figure 16.20 Stability Model Comparisons
The Reports section contains models fit by the Test Stability option. The following four models are fit by the Test Stability option:
• A different intercept, different slope model where the MSE (mean squared error) is pooled across batches. (If the Use Pooled MSE for Nonpoolable Model option is selected, this is the first model in the Stability Tests report.)
• A different intercept, common slope model. (This is the second model in Stability Tests report.)
• A common intercept, common slope model. (This is the third model in Stability Tests report.)
• A different intercept, different slope model where the MSE (mean squared error) is not pooled across batches. (If the Use Pooled MSE for Nonpoolable Model option is not selected, this is the first model in the Stability Tests report.)
Caution: In addition to the four models fit by the Test Stability option, the Reports section also contains any models fit in the Degradation platform prior to running the Test Stability option.