Nonparametric Test Reports
In the Oneway platform, there are nonparametric options for comparing means. This section covers reports for these methods.
The methods include testing the hypothesis of equal means or medians across groups. Nonparametric tests use functions of the response ranks, called rank scores. See Hajek (1969) and SAS Institute Inc. (2024a). Nonparametric multiple comparison procedures are also available to control the overall error rate for pairwise comparisons. See Nonparametric Multiple Comparisons Reports.
Wilcoxon Kruskal-Wallis, Median, Friedman Rank, and Van der Waerden Test Reports
For Wilcoxon Kruskal-Wallis, Median, Friedman Rank, or Van der Waerden Tests in the Oneway platform, two or three tables provide summary statistics and test results. The summary statistics tables contain the following columns:
Level
The levels of X.
Count
The frequencies of each level.
Score Sum
The sum of the rank score for each level.
Expected Score
The expected score under the null hypothesis that there is no difference among class levels.
Score Mean
The mean rank score for each level.
(Mean-Mean0)/Std0
The standardized score. Mean0 is the mean score expected under the null hypothesis. Std0 is the standard deviation of the score sum expected under the null hypothesis. The null hypothesis is that the group means or medians are equal across groups.
Two-Sample Test, Normal Approximation Table
When the X variable has exactly two levels, a 2-Sample Test, Normal Approximation table contains the following columns:
S
The sum of the rank scores for the level with the smaller number of observations.
Z
The test statistic for the normal approximation test that uses a 0.5 continuity correction. See Two-Sample Normal Approximations.
Prob>Z
The one-sided p-value for the normal approximation test that uses a 0.5 continuity correction. This p-value is based on a standard normal distribution.
Prob>|Z|
The p-value for the normal approximation test that uses a 0.5 continuity correction. This p-value is based on a standard normal distribution.
Note: In the Wilcoxon / Kruskal-Wallis Tests (Rank Sums) report, this table is called the Wilcoxon Two-Sample Test, Normal Approximation table.
One-Way Test, ChiSquare Approximation Table
The 1-Way (or Kruskal-Wallis) Test table contains the results of a chi-square test for location. See Conover (1999). If the number of groups is two, the Kruskal-Wallis test is equivalent to the Wilcoxon test.
ChiSquare
The value of the chi-square test statistic. See One-Way ChiSquare Approximations.
DF
The degrees of freedom for the test.
Prob>ChiSq
The p-value for the test. The p-value is based on a chi-square distribution with degrees of freedom equal to the number of levels of X minus 1. If the number of groups is two, this p-value is equal to the p-value for the normal approximation test without a 0.5 continuity correction.
Note: In the Wilcoxon / Kruskal-Wallis Tests (Rank Sums) report, this table is called the Kruskal-Wallis Test, ChiSquare Approximation table.
Friedman-Nemenyi Test
The Friedman Rank Test red triangle menu contains an option for the Nemenyi test. This test is a nonparametric version of the Tukey-Kramer multiple comparisons test. When the Friedman Rank Test is statistically significant, use the Nemenyi test to evaluate which pairs of items differs. The summary table contains the following columns:
Level
The first level of the X variable used in the pairwise comparison.
- Level
The second level of the X variable used in the pairwise comparison.
Score Mean Difference
The mean of the rank score of the observations in the first level (Level) minus the mean of the rank scores of the observations in the second level (-Level).
Z
The standardized test statistic, which has an asymptotic standard normal distribution under the null hypothesis of no difference in mean rank scores.
p-Value
The p-value for the asymptotic test based on Z.
Two additional sections of the report provide alternative summaries of the test results.
Pairwise P-value
A matrix of the pairwise p-values. These are the same values found in the summary table.
Connecting Letters Report
A traditional letter-coded table where levels that do not share a letter are significantly different.
Kolmogorov-Smirnov Two-Sample Test Report
In the Oneway platform, the Kolmogorov-Smirnov test report contains two tables: a summary table and an asymptotic test table. The summary table contains the following columns:
Level
The two levels of the X variable.
Count
The frequencies of each level.
EDF at Maximum
The value of the empirical cumulative distribution function (EDF) for that level of the X variable for which the difference between the two EDFs is a maximum. For the Total, the value is the pooled EDF (the EDF for the entire data set) at the value of the X variable for which the difference between the two EDFs is a maximum.
Deviation from Mean at Maximum
For each level, the value obtained by the following steps:
– Compute the difference between the EDF at Maximum for the given level and the EDF at maximum for the pooled data set (Total).
– Multiply this difference by the square root of the count of observations for that level.
The asymptotic test table contains the following columns:
KS
The Kolmogorov-Smirnov statistic computed as follows:
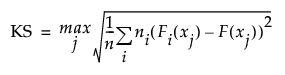
The formula uses the following notation:
– xj, j = 1,..., n are the observations
– ni is the number of observations in the ith level of X
– F is the pooled cumulative empirical distribution function
– Fi is the cumulative empirical distribution function for the ith level of X
Note: Although this version of the Kolmogorov-Smirnov statistic applies even when the X variable has more than two levels, in JMP the Kolmogorov-Smirnov option is available only when the X variable has exactly two levels.
KSa
The asymptotic Kolmogorov-Smirnov statistic computed as 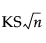 , where n is the total number of observations.
, where n is the total number of observations.
D=max|F1-F2|
The maximum absolute deviation between the EDFs for the two levels. This is the version of the Kolmogorov-Smirnov statistic typically used to compare two samples.
Prob > D
The p-value for the test. This is the probability that D exceeds the computed value under the null hypothesis of no difference between the levels.
D+ = max(F1-F2)
The one-sided test statistic for the alternative hypothesis that the level of the first group exceeds the level of the second group.
Prob > D+
The p-value for the test of D+.
D- = max(F2-F1)
The one-sided test statistic for the alternative hypothesis that the level of the second group exceeds the level of the first group
Prob > D-
The p-value for the test of D-.
Exact Test Reports
The reports for exact versions of nonparametric tests vary depending on the number of levels of the X variable. When the X variable has exactly two levels, the Oneway platform can perform an exact test for each nonparametric test type. For the Wilcoxon Kruskal -Wallis, Median, and Van der Waerden Exact Tests, the 2-Sample: Exact Test table contains the following columns:
S
The sum of the rank scores for the observations in the smaller group. If the two levels of the X variable have the same numbers of observations, then the value of S corresponds to the last level of the X variable in the value ordering.
Prob ≤ S
The one-sided p-value for the test. The direction of this test is determined by the sign of the difference in the two levels of the X variable.
Prob ≥ |S-Mean|
The two-sided p-value for the test.
Note: For the Kolmogorov-Smirnov exact test, the table provides the same statistics as the asymptotic test. However, the p-values are computed to be exact. See Kolmogorov-Smirnov Two-Sample Test Report.
When the X variable has more than two levels, the Oneway platform can perform an exact test for the Wilcoxon Kruskal -Wallis, Median, and Van der Waerden Tests. The ChiSquare Exact table contains the following columns:
ChiSquare
The value of the chi-square test statistic.
DF
The degrees of freedom for the test.
Prob>ChiSq
he p-value for the test.